​​The Use of Linear Perspective to Represent the Relationships in a Four-Dimensional Coordinate System

by

Randal J. Bishop

We associate images in linear perspective and sculptures with three-dimensional spaces.  There are four three-dimensional spaces in a four-dimensional coordinate system.  The preceding two sentences lead one to believe those two ideas would be extremely easy to put together, or have already been put together.  This paper will make the explanation and illustration of those ideas extremely easy because it will not require one to know all of the details about three and four-dimensional geometries. The first section will explain the principles and compositional elements (elements being points, lines and planes) of linear perspective.  The second section will explain the elements of two and three-dimensional Cartesian coordinate systems and our analogous associations with the elements of linear perspective and sculptures.  The third section will present the basic principles of dimensionality or multidimensional spaces while including a description with illustrations of a four-dimensional coordinate system.  The fourth section will present detailed explanations on how images in linear perspective and naturalistically scaled sculptural images can be used to represent the relationships in a four-dimensional coordinate system.

 

Section 1

 

The compositional devices known as linear perspective are familiar to those who have studied Western art.  In so many words, they are the principles guiding the compositional lines that allow for naturalistically scaled images in perspective.  Over the centuries, this phenomenon has been referred to as perspective, linear perspective, one-point perspective, three-point perspective, centric perspective, aesthetic perspective, scientific perspective, geometric perspective, mathematical perspective and skenographia. The easiest way to capture and exhibit an image in linear perspective is with a photograph. Basic naturalistic images in perspective can also be recreated with most aesthetic mediums: drawing, oil painting or any technique for creating a motion picture.  Now, a conventional description of an image in linear perspective will be followed with our analogous associations with a three-dimensional Cartesian coordinate system.

 

Leonardo da Vinci’s fresco, Last Supper, 1495-1498, is the most noted example of one-point perspective because its compositional lines are blatantly apparent and it still exists.  The compositional lines appear as the edges of the tapestries, walls, ceiling beams and table. When visually continued, these lines converge with the line of sight at the point of infinity (marked by the figure of Jesus) to create an accurate diagram of one-point perspective.  This line of sight is also perpendicular to the projected plane, in the middle ground, on which the figures of the apostles are arranged. This plane divides the space in front of the figures from the cavernous space of the room behind the figures. The relevant aspects of this composition are the following.  There is a line of sight (a one-dimensional line) perpendicular to a two-dimensional plane that can be seen as dividing the whole image. For more details and diagrams about pictorial compositions, see Martin Kemp’s book, The Science of Art.

This type of compositional structure naturally presents the appearance of a foreground, middle ground and background.  The usage of these terms in this paper will be limited to these very general and traditional definitions.  The foreground of an image contains the space in front of the projected plane in the middle ground and everything on the “picture plane”.  A “picture plane” is the whole surface the image is on, be it a wall or canvas, etc.  A “picture plane” is also referred to as the “window” through which the image is seen.  The middle ground shows where the line of sight perpendicularly intersects the projected plane on which most of the subject matter is placed.  The background is the space behind the foreground and the projected plane in the middle ground. 

Perspective was studied by many people during the Renaissance.  Leon Battista Alberti is credited for writing the first treatise on centric perspective entitled De Pictura, 1435.  The most authoritative and cited English translation to date is by Cecil Grayson entitled On Painting and On Sculpture. The origin of geometric perspective in art would not be complete without mentioning Alberti’s dedication in 1436 of his treatise to Filippo Brunelleschi. Brunelleschi is alleged, by his biographer Antonio di Tuccio Manetti, to have created the first two, now lost, paintings of scenes in perspective of Florence, Italy.  The exact dates of the panels are not known; however, in Samuel Y. Edgerton’s book, The Renaissance Rediscovery of Linear Perspective, Edgerton dates at least one of the panels to pre-1425 because of other paintings by Masaccio and Masolino dating from circa 1425-1427.  Edgerton’s research is reified with a photographic recreation of Brunelleschi’s first painting in perspective. The writings of William M. Ivins, On the Rationalization of Sight and particularly Art and Geometry, link the understandings various cultures throughout the ages had of optics and geometries to the developments of linear perspective and modern geometries. Irwin Panofsky’s book, Renaissance and Renascences in Western Art, thoroughly documents the aesthetic developments leading to accurately scaled, realistic images in perspective.  The Fourth Dimension and Non-Euclidean Geometry in Modern Art by Linda Dalrymple Henderson addresses more recent explorations between the arts and n-dimensional geometries. Clifford A. Pickford's amusing book Surfing Through Hyperspace: Understanding Higher Universes in Six Easy Lessons uses humor, something this subject really needs. Many great writers have documented the connectivity of art and geometric techniques during the past six centuries. 

 

Section 2

 

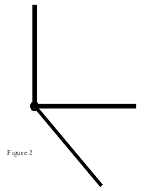
Today, it is common for us to say that images in perspective represent the third dimension or are two-dimensional representations of three-dimensional spaces. It is also common for us to say sculptures are three-dimensional.  These associations started after René Descartes developed what are now called two and three-dimensional Cartesian coordinate systems.  A two-dimensional coordinate system (Figure 1) is composed of two perpendicular lines and a three dimensional coordinate system (Figure 2) is composed of three mutually perpendicular lines. A three-dimensional Cartesian coordinate system is occasionally referred to as the X Y Z axes.

 

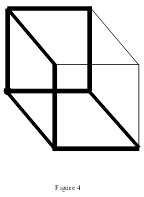
A two-dimensional coordinate system can become a square (Figure 3) and a three-dimensional coordinate system can become a cube (Figure 4) when their reference lines and reference planes are orthographically projected.  Orthographic projection means “moving” a reference line or plane to become a projection line or plane.  In the diagrams, the reference lines and planes are thicker than the projection lines and planes. Figure 5 illustrates a projection line (line ab) and projection planes (thin lines) in a three-dimensional coordinate system.  Projection line (line ab) is projected from and is parallel to reference line (line AB) while the two projection planes (thin lines) are projected from and are parallel to the reference plane at the back of the coordinate system.  This automatically makes projection line (line ab) perpendicular to the projection planes. The projection line (line ab) and the projection planes are analogous to a line of sight and planes in an image in perspective.  In an image in perspective a line of sight perpendicularly passes through the “picture plane” or “window” at the front of the image while it perpendicularly intersects a plane in the middle ground of the image.  The perpendicularity of these lines and planes remains consistent in three-dimensional coordinate systems and images in perspective.  This perpendicular consistency is the imperative factor to the introduction of, both, images in perspective and naturalistically scaled sculptural images into higher four-dimensional spaces.

 

Refer back to da Vinci’s Last Supper.  The composition of this fresco should allow one to see how photographic or two-dimensional naturalistic images in perspective can be used to represent the three-dimensional spaces in a four-dimensional space.  In another fresco (by Masaccio, entitled Trinity) an architectural like frame is painted around the “window” while two figures of people and an altar table with a tomb below are painted on the “picture plane” in front of the “window”.  This masterpiece, probably dating from the latter part of 1425, contains all of the compositional devices one needs to comprehend the introduction of photographic or two-dimensional naturalistic images in perspective and naturalistically scaled sculptural imagery into higher dimensional spaces.   

In summation, it is easy to see our analogous associations between the compositional elements of perspective and the geometric elements of three-dimensional geometry. This knowledge is actually a good introduction to dimensionality or multidimensional spaces and n-dimensional or higher dimensional geometries.

 

Section 3

 

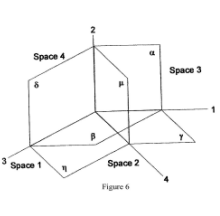
The concepts of dimensionality and higher dimensional spaces are easy to understand when introduced in the context of zero, one, two and three-dimensional spaces. These descriptions of some of the terminology of the discipline may help. A zero-dimensional space is called a vertex. A one-dimensional space is called a valence. Vertices and valences do not have volume. Vertices are zero-dimensional spaces that can be designated with letters, numbers or points. Valences are one-dimensional spaces, of any length, that can be designated with letters, numbers or lines. When two valences or one-dimensional spaces intersect at a vertex or zero-dimensional space they can form a two-dimensional space (i.e., a plane), and so on. The other dimensions are also composed of these geometric elements. A three-dimensional space occurs when three one-dimensional spaces intersect at a zero-dimensional space to form three two-dimensional spaces. A four-dimensional space occurs when four one-dimensional spaces intersect at a zero-dimensional space to form six two-dimensional planes and four three-dimensional spaces ( Figure 6).  That’s it, an extremely simplified description and illustration of the fourth dimension. The actual geometry is not that simple.  As a matter of fact, an unapologetic, irreverent over simplification of that magnitude would be an insult to all the mathematicians and geometers who have given us higher dimensional geometries. So, please allow for these notes. The four-dimensional coordinate system used in this paper has been referred to as a hypertetrahedron, four simplex and five-cell (the five-cells being the four three-dimensional cells while the fifth cell is regarded as the overall space encompassed by the four-dimensional coordinate system). The four-dimensional system in this paper is from the book ​Four-Dimensional Descriptive Geometry by C. Ernesto S. Lindgren and Steve M. Slaby. Thomas Banchoff’s most recent book, Beyond the Third Dimension is a comprehensive, easy-to-read introduction to dimensionality and higher dimensional geometries. The Fundamentals of Three-Dimensional Descriptive Geometry, by Steve M. Slaby, is an excellent introductory textbook on the subject.  Hypergraphics: Visualizing Complex Relationships in Art, Science and Technology, edited by David W. Brisson, was a pioneering anthology on these multidisciplinary issues.  The book Space Structures: Their Harmony and Counterpoint, by Arthur L. Loeb, and the academic journal HyperSpace are for those who would like to explore the vastness of these subjects. However, as mentioned in the beginning of this paper, all of the details about higher dimensional geometries and its century plus old history do not have to be known to understand this development. This was not the case with some of the details regarding linear perspective and three-dimensional geometry, which turned out to be a good introduction to four-dimensional geometry.

We only have to know some of the geometry’s basic notions to understand this development.  All of the geometry being referred to is from the book Four-Dimensional Descriptive Geometry by C. Ernesto S. Lindgren and Steve M. Slaby.  The basic notions being referred to are called the conditions of perpendicularity. Those conditions are four statements specifying the perpendicular relationships of the four reference lines forming the six two-dimensional planes and four three-dimensional spaces in a four-dimensional coordinate system. The following four requirements for a four-dimensional coordinate system, from page 22 in their book, are similar to the mutually perpendicular requirements of the three lines and three planes in a three dimensional Cartesian coordinate system.

 

1. The four spaces of the system, taken in threes, determine the four lines that are perpendicular to each other and belong to the same point.

2. Four lines, taken in threes, determine the four spaces that are perpendicular to each other.

3. Four lines, taken in pairs, determine six planes, which, taken in threes, form four groups of planes belonging to the same line and are mutually perpendicular.

4. Any one line of the reference system is perpendicular to a space determined by the other three lines in the system.

 

These four statements provide a verbal description of a four-dimensional coordinate system.  While Figure 6, from page 17 in their book, as a diagram provides only a visual indication of the conditions of belonging and not the conditions of perpendicularity. In other words, it just shows the lines belonging to certain planes and spaces, not the perpendicular relationships among the lines, planes and spaces. (Note: the lines have been
given numbers and the planes have been given lower-case Greek letters).

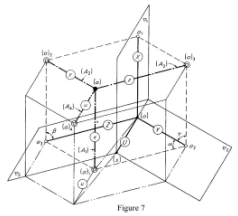
When their book was published in 1968, Lindgren and Slaby used Figure 7, amongst many others, to illustrate all of the perpendicular alignments of the elements in a four-dimensional coordinate system.  Their publication still stands as the definitive treatise on four-dimensional descriptive geometry. 

Luckily, there is a way to show everything in those diagrams using four images or sculptures of natural people standing on natural ground to indicate the proper relationships of the three-dimensional spaces in a four-dimensional space.

 

Section 4

This can be achieved by applying the conditions of belonging and perpendicularity to four lines of sight, just as they can be applied to four reference lines.  This does not just mean the images of spaces in perspective and naturalistically scaled sculptural images must be placed in the correct three-dimensional space in the four-dimensional system – meaning placing the image of space 1 in space 1 in the coordinate system. It means the lines of sight with accompanying images must visually belong to certain lines, planes and spaces in the system while being perpendicular to other lines, planes and spaces in the system.

Keep in mind, when dealing with realistic imagery two sets of aesthetic issues have to be addressed to present all of the relationships of the lines, planes and spaces inside of a four-dimensional coordinate system. The first aesthetic issue involves compositional lines and planes of an image in perspective; each of the four images should have obvious foregrounds, middle grounds and backgrounds and unobstructed lines of sight.  The second issue involves the visual distinctiveness of the subjects or figures in the images; each one of the four images should contain four visually distinct subjects or figures so as to be readily identifiable with one of the four distinct spaces.  Each of these aesthetic issues should complement the other.  Consequently, the compositional elements of the images must not only belong to the corresponding elements of the spaces in the four-dimensional coordinate system, the lines of sight in each image (just like the reference lines) must clearly be perpendicular to the correct three-dimensional space inside of the four-dimensional coordinate system, and the subjects or figures in the images should also show in their own backgrounds an image of the space it must be perpendicular to in the four-dimensional coordinate system. In this way the introduction of naturalistic imagery adds extra requirements that need to comply with the requirements of the geometry. However surprisingly, the end result of this complex compilation will be works of art free of obvious geometric notations  (notations being letters, numbers or lines) that readily exhibit all of the aesthetic and geometric aspects of this amalgamation.

 

These four images can be derived from three points in opposition (meaning three points in a row).  The two images taken from the two outer points must be directed toward the central point, while the other two images taken from the central point must be directed toward the outer points. This pattern establishes a direction for the lines of sight that correspond to the needs of the reference lines to intersect some of the other reference lines at perpendicular angles.  In an effort to ingest as little as possible let these reminders suffice.  Lines of sight can be interpreted as emanating from the viewer or from the subject (see William M. Ivins’ writings on the study of optics during the Middle Ages).  Now back to the assimilation of the images to the geometry.  Each of these images of spaces must simultaneously represent a line, plane and three-dimensional space within a four-dimensional coordinate system. This is achieved when the spaces are numbered (from left to right) 1,2,3,4 and respectively designated with reference or projection planes µ, η, α, ß and reference or projection lines 3, 1, 4 and 2. Planes γ and δ, the other two planes in the system, must be mutually perpendicular to planes µ, η, α  and ß. These relationships occur when plane γ shows the ground or horizontal plane of spaces 2 and 3, and plane δ shows the ground or horizontal plane of spaces 1 and 4. The side of viewing the spaces between the three points in opposition does not matter or if the photographs are numbered from right to left, as long as space 1 is associated with plane µ and line 3, space 2 with plane η and line 1, space 3 with plane α and line 4 and space 4 with plane ß and line 2; the geometry is composed of reciprocal statements therefore the result will be the same four arrangements. The reciprocal nature of this geometry allows for, at least, these two aesthetic interpretations of any space chosen to illustrate a four-dimensional coordinate system.

 

When choosing a natural space remember each image should obviously appear to belong to the same natural space, while maintaining a distinct subject or figure in each one of the four spaces. In the four three-dimensional spaces used to illustrate the system described in this paper, there is a car to the far left, in the middle of space 1, a man in the middle of space 2, a woman in the middle of space 3 and a tree in the middle of space 4.

The alignment of these four figures in their natural spaces can be seen during the first 15 seconds of the computer graphics. It is aesthetically relevant to note the slightly staggered from center alignments of the man, woman and tree to allow for unobstructed lines of sight through the four spaces.

 

The first few seconds of the graphics shows a fly through to the central point in the natural space. This is also the image of space 3 containing the figure of the woman in space 3 and the diminutively scaled figure of the tree in the background on reference plane α; the tree is actually in space 4.  At this central point in the natural space the camera turns to face and fly towards the same image in the four-dimensional coordinate system. As the computer’s camera enters space 3 in the system, it follows the line of sight, parallel to reference line 4, through space 3 to ultimately be perpendicular to space 4 with the tree in it.

 

The precise perpendicular relationships are not illustrated due to limitations regarding the computer graphics. The figure of the man is facing the wrong direction in space 2; it should be turned 180° to face the direction the line-of-sight or reference line must emanate from so the lines can ultimately be perpendicular to space 1.  Nevertheless, if reference line 4 was made perpendicular to reference line 1 half of the conditions of perpendicularity would be satisfied; then if reference line 3 was made perpendicular to reference line 2 the other half of the conditions of perpendicularity would be satisfied.

 

The results of these two arrangements will be the following: reference line 4 will belong to spaces 1, 2, 3 and plains γ, η, µ while being perpendicular to space 4, reference line 1 will belong to spaces 2, 3, 4 and plains α, ß, γ while being perpendicular to space 1, reference line 3 will belong to spaces 1, 2, 4 and plains ß, δ, η while being perpendicular to space 3, reference line 2 will belong to spaces 1, 3, 4 and plains α, δ, µ will being perpendicular to space 2.

Different arrangements of the four fluctuating reference lines would provide aesthetically correct variations of the geometric requirements. In regards to the fly through of space 3, when reference lines 1 and 2 are simultaneously perpendicular to reference line 4 an isometric cube or space would form.  The naturalistically scaled sculptural image of the woman in space 3 would not be subject to diminution because the sculptural imagery in the space must be available for 360° inspection, just as it was in the natural space.  At this point, it would become aesthetically viable to have a diminutively scaled image of the background of space 3 (which is the tree in space 4) on reference plain α. This would provide another aesthetic presentation of the perpendicular relationships of reference line 4 to space 4. The same aesthetized arrangements of the reference lines could also be applied to the reference lines forming the other spaces. 

The rediscovery of linear perspective during the Renaissance inspired us to classify and categorize spaces and structures using geometric techniques, which eventually provided us with definitions of two and three-dimensional spaces.  Relatively new geometries, from the latter part of the nineteenth and twentieth centuries, have provided us with definitions of higher dimensional spaces and structures, including four-dimensional spaces and structures and many others.  Finally, naturalistic imagery can be infused into kinetic four-dimensional sculptures and spaces.  These new works of art will allow us to plainly see and understand the long mutually beneficial evolutionary relationship between art and geometry.